Tip 1: Order Does Not Matter
When we multiply two numbers, it does not matter which is first or second, the answer is always the same.
Example: 3×5=15, and 5×3=15
Another Example: 2×9=18, and 9×2=18
In fact half of the table is a mirror image of the other!
So, don't memorize both "3×5" and "5×3", just memorize that "a 3 and a 5 make 15" when multiplied.
This is very important! It nearly cuts the whole job in half.

In your mind you should think of
3 and 5 "together" making 15.
so you should be thinking something like this:

Tip 2: Learn the Tables in "Chunks"
It is too hard to put the whole table into your memory at once. So, learn it in "chunks" ...
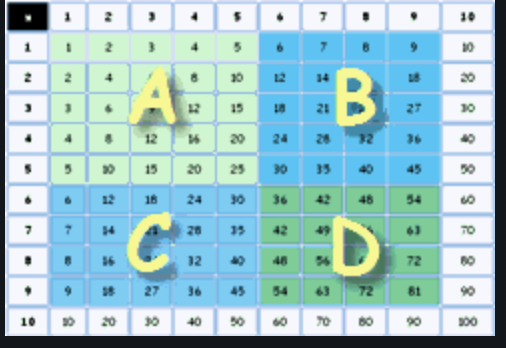
- A Start by learning the 5 times table.
- B Then learn up to 9 times 5.
- C Is the same as B, except the questions are the other way around. Learn it too.
- D Lastly learn the "6×6 to 9×9" chunk
Then bring it all together by practicing the whole "10 Times Table"
And you will know your 10 Times Table!
(We look at the 12x table below)
Some Patterns
There are some patterns which can help you remember:
2× is just doubling the number. The same as adding the number to itself.
2×2=4, 2×3=6, 2×4=8, etc.
So the pattern is 2, 4, 6, 8, 10, 12, 14, 16, 18, 20
(And once you remember those, you also know 3×2, 4×2, 5×2, etc., right?)
5× has a pattern: 5, 10, 15, 20, etc. It ends in either 0 or 5.
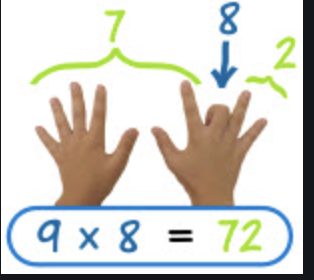
9× has a pattern, too: 9, 18, 27, 36, 45, 54, 63, 72, 81, 90
Now, notice how the "ones" place goes down: 9, 8, 7, 6, ...? And at the same time, the "tens" place goes up: 1, 2, 3,...? Well, your hands can help!
Example: to multiply 9 by 8: hold your 8th finger down, and you can count "7" and "2" ... the answer is 72
10× is maybe the easiest of them all ... just put a zero after it.
10×2=20, 10×3=30, 10×4=40, etc.
What About the 12 Times Table?
Sounds tough, but once you have mastered the 10× table, it is just a few steps away.
Firstly, 11× is mostly easy: from 11×2 to 11×9 you just put the two digits together. 11×2=22, 11×3=33, ..., 11×9=99.
And of course 2×, 5× and 10× just follow their simple rules you know already.
So it just leaves these to remember:
- 3×12=36,
- 4×12=48,
- 6×12=72,
- 7×12=84,
- 8×12=96,
- 9×12=108
And the "Big 3":
- 11×11=121,
- 11×12=132 and
- 12×12=144
Mastering Multiplication with an Interactive Multiplication Chart
What is a Multiplication Chart?
A Multiplication Chart is an essential tool for learning and practicing multiplication. It's a grid that displays the products of multiplying numbers, typically from 1 to 12. This visual aid helps students understand multiplication patterns and relationships between numbers.
The Importance of a Multiplication Chart
A Multiplication Chart serves as a cornerstone for mathematical learning. It provides a clear, visual representation of multiplication facts, making it easier for students to grasp and memorize these fundamental concepts. By using a Multiplication Chart, learners can:
- Quickly find the product of two numbers
- Identify patterns in multiplication
- Develop a deeper understanding of number relationships
- Build confidence in their math skills
How to Use a Multiplication Chart
Using a Multiplication Chart is straightforward. Here's a step-by-step guide:
- Locate the first number you want to multiply in the left column of the multiplication chart.
- Find the second number in the top row of the multiplication chart.
- Trace these two numbers to where they intersect on the multiplication chart.
- The number at the intersection is your product.
For example, to find 6 x 7 on the Multiplication Chart:
- Locate 6 in the left column of the multiplication chart
- Find 7 in the top row of the multiplication chart
- The intersection shows 42, which is the product of 6 x 7
Interactive Features of Our Multiplication Chart
Our interactive Multiplication Chart offers several features to enhance the learning experience:
- Hover over any cell to highlight the corresponding row and column
- See the multiplication equation displayed when selecting a cell with multiplication chart
- Practice mode to test your multiplication skills with multiplication chart
- Customizable multiplication chart size (up to 12x12)
Benefits of Using a Multiplication Chart
Visual Learning Aid
A Multiplication Chart provides a visual representation of multiplication facts. This visual aid is particularly helpful for students who learn best through seeing and interacting with information. The chart allows learners to visualize patterns and relationships between numbers, making multiplication more intuitive.
Pattern Recognition
One of the key benefits of a Multiplication Chart is its ability to showcase multiplication patterns. Students can observe how products change as they move across rows or down columns. These patterns help in understanding the commutative property of multiplication and can lead to faster mental calculations.
Building Number Sense
Regular use of a Multiplication Chart helps develop a strong number sense. Students begin to understand the relationships between numbers and can estimate products more accurately. This foundational skill is crucial for more advanced mathematical concepts.
Confidence Booster
As students become more familiar with the Multiplication Chart, their confidence in handling multiplication problems grows. This increased confidence can lead to improved performance in math overall.
Strategies for Mastering the Multiplication Chart
Start Small
Begin with a smaller Multiplication Chart, such as a 5x5 grid. Master these basic facts before moving on to larger numbers. This approach builds confidence and ensures a solid foundation.
Look for Patterns
Encourage students to identify patterns within the Multiplication Chart. For example:
- Multiplying by 2 doubles the number
- Multiplying by 5 results in products ending in 0 or 5
- Multiplying by 9 has a pattern in the sum of its digits
Use Skip Counting
Practice skip counting along rows and columns of the Multiplication Chart. This reinforces multiplication as repeated addition and helps in memorizing multiplication facts.
Focus on Commutative Property
Highlight how 3 x 4 and 4 x 3 both equal 12 on the Multiplication Chart. Understanding this property effectively halves the number of facts to memorize.
Beyond Basic Multiplication chart
Extending to Larger Numbers
Once comfortable with the basic Multiplication Chart, extend learning to larger numbers. Discuss how to use the multiplication chart for multiplying two-digit numbers or beyond.
Introducing Division
The Multiplication Chart can also be used to understand division. Show how division is the inverse of multiplication using the chart as a reference.
Exploring Factors and Multiples
Use the Multiplication Chart to identify factors and multiples of numbers. This lays the groundwork for understanding more advanced concepts like prime factorization and least common multiples.
Incorporating the Multiplication Chart in Daily Learning
Regular Practice
Encourage daily practice with the Multiplication Chart. Even a few minutes each day can significantly improve multiplication skills over time.
Games and Challenges
Create games and challenges using the Multiplication Chart. For example:
- Multiplication bingo
- Speed drills to find products
- Scavenger hunts for specific products on the multiplication chart
Real-world Applications
Discuss how multiplication chart is used in everyday life. Use examples like calculating grocery costs or determining area to make the learning relevant.
Conclusion
A Multiplication Chart is more than just a learning tool; it's a gateway to mathematical understanding. By mastering this fundamental concept, students build a strong foundation for more advanced mathematical skills. Our interactive Multiplication Chart provides an engaging way to explore, learn, and practice multiplication facts. Whether you're a student, teacher, or parent, incorporating this powerful tool into your learning routine can lead to significant improvements in mathematical proficiency and confidence.
Remember, the journey to multiplication mastery is unique for each learner. Use the Multiplication Chart as a guide, but encourage exploration and discovery. With regular practice and the right approach, anyone can become a multiplication expert!
